The time constant is a crucial concept in electrical engineering, especially when dealing with inductors. Understanding how to calculate the time constant for an inductor with a specific inductance value is essential for both theoretical and practical applications in circuits. In this article, we will explore the relationship between inductance and the time constant, using an inductor with a value of 60.0 mH as our reference. We will break down the calculations, provide examples, and discuss the implications of the time constant in various electrical scenarios.
Inductance, measured in henries (H), quantifies the ability of an inductor to store energy in a magnetic field when electrical current passes through it. The time constant, denoted by the Greek letter tau (τ), represents the time required for the current to reach approximately 63.2% of its maximum value after a voltage is applied across the inductor. This concept is vital in understanding the behavior of inductors in RL circuits (resistor-inductor circuits).
Throughout this article, we will calculate the time constant for an inductor with a given inductance of 60.0 mH, explain the factors that affect the time constant, and provide insights into practical applications and scenarios where this knowledge is invaluable. Whether you are a student, engineer, or simply curious about electrical engineering concepts, this comprehensive guide will help you grasp the importance of the time constant in inductors.
Table of Contents
Understanding Inductance
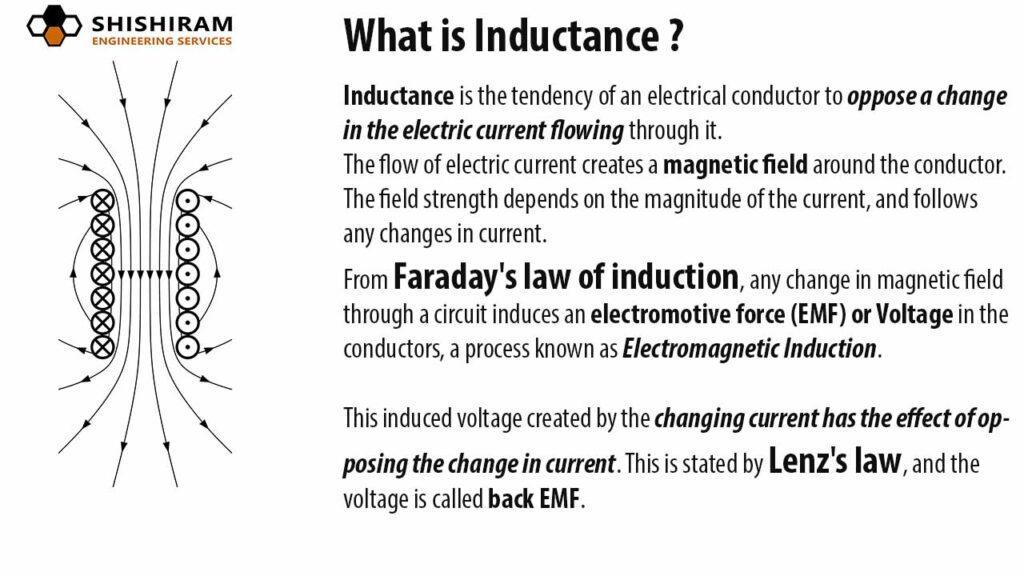
Inductance is a fundamental property of electrical circuits, especially in devices known as inductors. An inductor is essentially a coil of wire that creates a magnetic field when current flows through it. The inductance value indicates how much magnetic energy the inductor can store. It is measured in henries (H), and a common value for smaller inductors is in millihenries (mH).
What is Inductance?
Inductance (L) is defined as the ratio of the induced electromotive force (EMF) in a circuit to the rate of change of current flowing through it. The formula for calculating inductance is:
L = (NΦ) / I
Where:
- L = inductance in henries (H)
- N = number of turns in the coil
- Φ = magnetic flux in webers (Wb)
- I = current in amperes (A)
What is Time Constant?
The time constant (τ) for an inductor in an RL circuit is a measure of the time it takes for the current to reach approximately 63.2% of its maximum value after a voltage is applied. It is calculated using the formula:
τ = L / R
Where:
- τ = time constant in seconds (s)
- L = inductance in henries (H)
- R = resistance in ohms (Ω)
Calculating Time Constant
To calculate the time constant for an inductor with an inductance of 60.0 mH, we first need to convert the inductance from millihenries to henries:
60.0 mH = 60.0 x 10^-3 H = 0.060 H
Next, we need the resistance value (R) of the circuit to complete the calculation. Let's assume a resistance of 10 Ω for this example:
Now we can apply the formula for time constant:
τ = L / R = 0.060 H / 10 Ω = 0.006 s
This means the time constant for the inductor with 60.0 mH inductance and 10 Ω resistance is 0.006 seconds, or 6 milliseconds.
Factors Affecting Time Constant
Several factors can influence the time constant of an RL circuit:
- Inductance (L): Higher inductance results in a longer time constant, meaning the current takes more time to reach its maximum value.
- Resistance (R): Higher resistance reduces the time constant, allowing the current to rise more quickly.
- Initial Current: The starting current in the circuit can affect how quickly the current reaches its maximum value.
Practical Applications of Time Constant
The time constant is a critical parameter in various applications, including:
- Power Supplies: Understanding the time constant helps in designing efficient power supply circuits.
- Signal Processing: Time constants are used in filters and signal processing circuits to control the speed of signal changes.
- Control Systems: The time constant aids in designing control systems that respond appropriately to changes in input signals.
Examples of Time Constant in RL Circuits
Let’s explore a few examples to illustrate the calculation of time constants in RL circuits:
Example 1: Inductor with 100 mH and 20 Ω
Given: L = 100 mH = 0.1 H, R = 20 Ω
Calculation: τ = L / R = 0.1 H / 20 Ω = 0.005 s (5 milliseconds)
Example 2: Inductor with 200 mH and 50 Ω
Given: L = 200 mH = 0.2 H, R = 50 Ω
Calculation: τ = L / R = 0.2 H / 50 Ω = 0.004 s (4 milliseconds)
Summary
In summary, the time constant (τ) is a vital parameter in electrical engineering that helps us understand the behavior of inductors in circuits. By calculating τ for an inductor with an inductance of 60.0 mH and a given resistance, we were able to derive a time constant of 0.006 seconds. Factors such as inductance and resistance play a significant role in determining the time constant, which has various practical applications in power supplies, signal processing, and control systems.
Conclusion
Understanding the time constant for inductors is essential for anyone involved in electrical engineering or circuit design. We encourage readers to experiment with different inductance and resistance values to see how they affect the time constant in RL circuits. If you found this article helpful, please leave a comment, share it with others, or explore more articles on our site for a deeper understanding of electrical concepts.
ncG1vNJzZmivp6x7rLHLpbCmp5%2Bnsm%2BvzqZmm6efqMFuxc6uqWarlaR8qrKMrZ%2BeZZmjsbav06ipZqCRqHqqusOumq2ZnpiyboKPaWSmoF2staLAjKKqZqyYmnq1tcyeZJynnqjBorrTZp2oql2ptaq%2FjaGrpqQ%3D